Mosaic and Tile Number
The diagrams below are two illustrations of the knot 9_10. Each is built from small tiles of five types: one is blank, two have a single arc joining points on the boundary (ether adjacent or opposite), and two have two arcs joining pairs of points on the boundary (either adjacent or opposite with an over/under crossing). The tiles can be rotated in building the overall "mosaic."
It can be proved that for 9_10 the smallest square mosaic diagram is 6 by 6. Thus, its mosaic number is 6. It used 32 nonempty tiles. The figure on the right has 27 nonempty tiles, and this is the minimum for the knot 9_10. Thus, it has tile number 27.
For much more information, visit Aaron Heap's website Knot Mosaic Space.
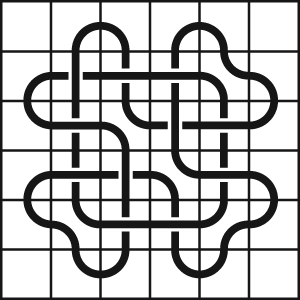 |
 |
| Minimal Mosaic Number | Minimal Tile Number |
Specific Knots
References
[1] Adams, C.; Flapan, E.; Henrich, A.; Kauffman, L.; Ludwig, L.; Nelson, S. Encyclopedia of Knot Theory, 1st ed., Chapman and Hall/CRC, 2021.
[2] Heap, A.; Knowles, D. Tile Number and Space-Efficient Knot Mosaics; J. Knot Theory Ramif. 2018, Vol. 27, Issue 6. Heap, A.; Knowles, D. Space-Efficient Knot Mosaics for Prime Knots with Mosaic Number 6; Involve 2019, Vol. 12, Issue 5.
[3] Heap, A.; LaCourt, N. Space-Efficient Prime Knot 7-Mosaics; Symmetry 2020, Vol. 12, Issue 4.
[4] Heap, A.; Baldwin, D.; Canning, J.; Vinal, G. Tabulating Knot Mosaics: Crossing Number 10 or Less; in preparation.
[5] Howards, H.; Kobin, A. Crossing Number Bounds in Knot Mosaics; J. Knot Theory Ramif. 2018, Vol. 27, Issue 10.
[6] Kuriya, T.; Shehab, O. The Lomonaco–Kauffman Conjecture; J. Knot Theory Ramif. 2014, Vol. 23, Issue 1.
[7] Lee, H.; Hong, K.; Lee, H.; Oh, S. Mosaic Number of Knots; J. Knot Theory Ramif. 2014, Vol. 23, Issue 13.
[8] Lee, H.; Ludwig, L.; Paat, J.; Peiffer, A. Knot Mosaic Tabulation; Involve 2018, Vol. 11, Issue 1.
[9] Lomonaco, S.J.; Kauffman, L.H. Quantum Knots and Mosaics; Quantum Inf. Process. 2008, 7, 85–115.
[10] Ludwig, L.; Evans, E.; Paat, J. An Infinite Family of Knots Whose Mosaic Number Is Realized in Non-reduced Projections; J. Knot Theory Ramif. 2013, Vol. 22, Issue 7.